Computational Fluid Dynamics (CFD) in Ophthalmology

From EyeWiki

All content on Eyewiki is protected by copyright law and the Terms of Service. This content may not be reproduced, copied, or put into any artificial intelligence program, including large language and generative AI models, without permission from the Academy.

Article initiated by:
Konstantinos Tsaousis, MD
All contributors:
Ahmad A. Aref, MD, MBA, Daniel B. Moore, MD, Konstantinos Tsaousis, MD, Ian Conner, MD, PhD, Abdelrahman M. Elhusseiny, MD, MSc, Mark Slabaugh, MD
Assigned editor:
Mark Slabaugh, MD
Review:
Assigned status Up to Date
 by Mark Slabaugh, MD on November 15, 2024.


Preface

The aim of this mini review is to present some efforts for application of computational fluid dynamics in the science of Ophthalmology. It is more than apparent that the eye itself, comprises a brilliant model for this kind of studies, in view of a possible clinical application in the near or not so near future.

Introductory knowledge

Definitions

A fluid is a substance that deforms under an applied shear stress. Fluids are a subset of the phases of matter and include liquids, gases, plasmas, and plastic solids. They can be defined as substances that have zero shear modulus or in simpler terms a fluid is a substance which cannot resist any shear force applied to it.

Depending on the relationship between shear stress, and the rate of strain and its derivatives, fluids can be characterized as one of the following:

  • Newtonian fluids : where stress is directly proportional to rate of strain
  • Non-Newtonian fluids : where stress is not proportional to rate of strain, its higher powers and derivatives.

The behavior of fluids can be described by the Navier–Stokes equations—a set of partial differential equations.

Figure 1: Example of computational fluid dynamics in a physical phenomenon (water wave propagation)

The human body is characterized by a high concentration of fluids so a reasonable thought is to describe certain functions with the assistance of computational fluid dynamics (CFD). Computational fluid dynamics is one of the techniques of fluid mechanics that uses numerical methods and algorithms to investigate and solve problems that involve fluid flow.[1] Using CFD, one can assemble a computational model that represents a structure. Application of the fluid flow in physics and chemistry to this virtual model will yield a guess of the fluid dynamics and related physical incidents (Figure 1).

Consequently, CFD is a refined computationally-based technique for analysis. CFD software gives the ability to imitate flows of gases and liquids through computer modeling. The elementary starting point of almost all CFD problems is the Navier–Stokes equations, which characterize any single-phase fluid flow. These equations can become less complicated by eradicating terms relating viscosity to give up the Euler equations. To make things even more straightforward, by removing terms describing vorticity the full potential equations can be extracted. In conclusion, these equations can be linearized to yield the linearized potential equations.[2]

It is a widespread belief amid visual researchers that solutions to scientific and even clinical problems unsolved until today, could be achieved through intense interaction and cooperation between scientists of diverse scientific backgrounds. Towards this direction many multidisciplinary academic programs and scientific projects are organized in order to establish a better base of understanding between medical doctors, mathematicians, physicists etc.

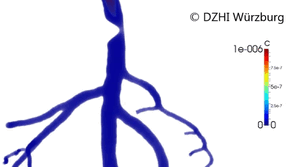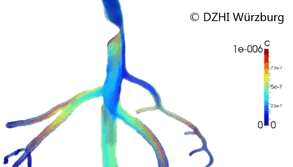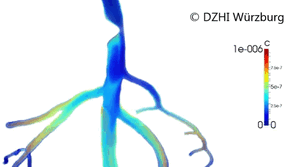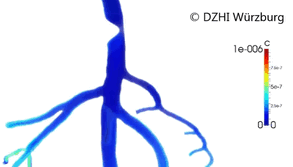
This type of approach has provided valuable outcomes regarding issues in cardiology such as atherogenesis[3] or artificial implants performance (stents).[4][5] Computer modeling of the blood vessels is considered to have the potential to drastically alter 21st century cardiovascular research (Figure 2).

Figure 2: Simulation of blood flow within the aortic system.

During the last years, lots of efforts are attempted to connect the CFD science with other fields of medicine including ophthalmology.[6][7][8]

Ophthalmology

In ophthalmology, CFD can easily applied for the investigation of aqueous humor and vitreous body behavior and partially crystalline lens.[9][10] The above mentioned structures enforce eye’s stability keeping and in addition are involved in pathologic conditions such as glaucoma and vitreoretinal disorders.

Aqueous humor - Glaucoma

Physiology

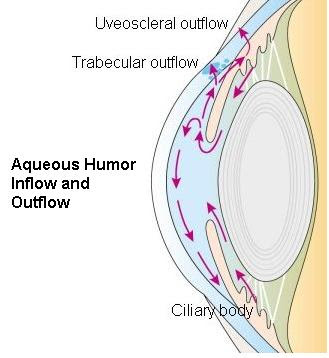
Table 1. Production and drainage of the aqueous humor
Aqueous humour is secreted into the posterior chamber by the ciliary body, specifically the non-pigmented epithelium which is located in the pars plicata.
↓
It flows through the wiry opening between the front of the lens and the back of the iris, to run off through the pupil into the anterior chamber, and then to drain out of the eye via the trabecular meshwork.
↓
From there, it drains into Schlemm's canal by one of two ways: directly, via aqueous vein to the episcleral vein, or indirectly, via collector channels to the episcleral vein by intrascleral plexus and in due course into the veins of the orbit.
Figure 3. Aqueous humor circulation

Aqueous humor is constantly produced and this pace of production should be balanced by an equivalent rate of drainage. Little variations in the production or outflow of aqueous humor will have a great impact on the intraocular pressure (IOP). The maximum resistance to aqueous flow is provided by the trabecular meshwork (TM), and this is where the majority of the aqueous outflow occurs.

The secondary route is the uveoscleral one, and is independent of the intraocular pressure, the aqueous flows through here, but to a minor degree than through the TM.

Pathophysiology

There is a number of queries regarding the aqueous humor that remain intricate to understand. An exceptional paradigm is glaucoma pathophysiology.[11]

Glaucoma is unique between the ophthalmic diseases because its clinical management and basic pathophysiology requires comprehension of ocular structures of both the anterior and posterior segment.[12] In the normal eye, there is a fragile balance between the inflow and outflow of aqueous.[13] When the outflow is blocked, the IOP increases, causing optic nerve damage.[14] This process is recognized as glaucoma. Even if the IOP was excluded of the definition of glaucoma, it should remain as a causal risk factor for the development and progression of glaucoma. Increased IOP is related with increased resistance to aqueous humor outflow, whilst the rate of aqueous humor formation is no different from that in normal (non-glaucomatous) individuals.[15][16]

These remarks led to the call for for more deep understanding of aqueous humor properties and the inexorable support of CFD.

Figure 4: Natural Flow and Thermal Phenomena, typically involving buoyancy-driven flow

Computational modeling

With regards to several parameters of the human eye, researchers attempted to present the characteristics of aqueous humor out flow in the anterior chamber of the eye.[17] They concluded that the physical mechanisms responsible for causing such flows may be classified as follows:

1 Buoyancy-driven (natural convection) flow arising from the temperature difference between the anterior surface of the cornea and the iris (Figure 3)
2 Flow generated by the aqueous production of the ciliary body
3 Flow generated by the interaction between buoyancy and gravity while sleeping in a face-up position
4 Flow generated by phacodonesis (lens tremor)
5 Flow generated by Rapid Eye Movement (REM) during sleep

Each flow is studied using a traditional fluid mechanics/asymptotic analysis approach.

A variety of useful results and perhaps clinical tools could be retrieved such as:

  • The shear stress may be calculated to determine whether or not the flow is strong enough to detach pigment particles from the iris. Comparison with the experimental values of Gerlach et al. (1997) and Vankooten et al. (1994) have shown that the flow is not nearly powerful enough to cause such effects, so that buoyancy-driven flow in the anterior chamber cannot be solely responsible (as has previously been suggested) for the presence of pigment particles in the anterior chamber.
  • Predictions may be made for hyphemas (blood in the anterior chamber), hypopyons (white blood cells) and Krukenberg spindles (pigment particles).
  • The buoyancy induced by temperature gradients in the eye is by far the most pervasive mechanism for causing anterior chamber flow, producing velocities that are many orders of magnitude superior than those attributable to any further physical mechanism.

For the realization of the particular study the scientists used the FEMLAB©, a commercial limited element equation solver, solving the full Navier–Stokes equations and corresponding total coupled convection/diffusion problem.

  • Researchers tried to make a mathematic model about ocular fluid dynamics in a rabbit eye.[18] The calculations are based on a geometrical model of the eye, which represents the TM as a multilayered porous zone of specified pore sizes.. Variations in the pupil size shows to have modest influence on the IOP or flow distribution in view of the dominant role of buoyancy in controlling the flow motion. The study provides distributions of the shear stress and flow patterns and outlines the imperative role of the eye-orientation on these results.
Figure 5. Cofiguration of iris in open and narrow angles
  • Analogous effort focused on modeling passive mechanical interaction between aqueous humor and iris. A mathematical model of the coupled aqueous humor–iris system accounts for the contribution of aqueous humor flow and passive iris deformability to the iris contour, presented.[19] The aqueous humor is modeled as a Newtonian fluid, and the iris is modeled as a linear elastic solid. The model was used to calculate the iris contour in healthy and diseased eyes.

The results compare favorably with clinical observations, supporting the hypothesis that passive iris deformation can produce the iris contours observed using ultrasound biomicroscopy. In order to develop the model, researchers calculated a maximum Reynolds number of 0.01 in the aqueous humor under steady-state conditions, consistent with Friedland’s earlier estimate. However, in transient phenomena, such as blinking, the rapid movement of the boundaries causes the acceleration of the fluid to become significant, requiring the use of the full Navier–Stokes equations. Experiments on the bovine iris have shown that the tissue is incompressible and linearly elastic under small deformations with a Young’s modulus, E, of 27 kPa in the radial direction. These measurements support the use of the incompressible linear elastic equations to model the iris. Although the supposed rest position of the iris is on the crystalline lens, hydrodynamics of the aqueous humor force the iris away from the lens surface.

  • One more model has been proposed to explain aqueous humor hydrodynamics in human eye heat transfer.[20] The need to develop accurate representation of the human eye for the purpose of physiological studies is important to ensure that the predicted results are reliable. The presence of natural circulation of aqueous humor is evident from clinical, experimental, and simulated observations. Most of the thermal models of the human eye that are found in the literature, however, had assumed an inactive aqueous humor inside the anterior chamber. In this study, a two-dimensional model of the human eye is developed where the circulation of aqueous humor inside the anterior chamber is included. The effects of the aqueous humor flow on the temperature allocation inside the eye are investigated. The natural circulation of aqueous humor was found to increase the temperature and alter the temperature profile of the cornea and anterior chamber.

A lot of attention gathered the function of TM in the flow of aqueous humor.

Laser iridotomy

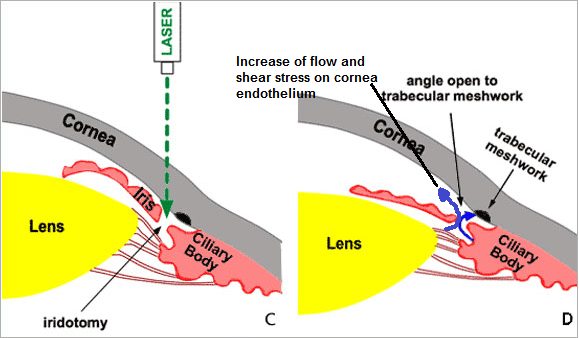
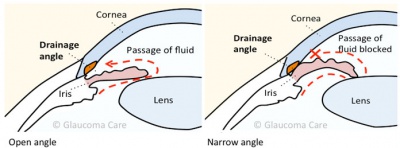
Figure 6. Increase of aqueous humor flow and shear stress in the anterior chamber after laser PI (peripheral iridotomy)

A model has been proposed to predict flow in Schlemm’s canal and wall shear stresses in Schlemm’s canal.[21] As regards shear stress, it emerges to have significant role in attachment of corneal endothelial cells. Computational fluid dynamics tried to investigate is the effect of anterior chamber depth on shear stress exerted on corneal endothelial cells by altered aqueous flow after laser iridotomy.[22] The study hypothesis was that shear stress caused by abnormal aqueous flow is one of the causes of corneal endothelial cell loss after laser iridotomy (LI). (Figure 6)The shear stress exerted on the corneal endothelial cells (CECs) in anterior chambers of different depths was calculated by a CFD program.

The effect of shear stress was also examined on human CECs grown on microscope slides. Conclusion of the study was that shear stress exerted on human CECs after LI may reach a scale adequate to cause cell damage and loss in eyes, especially in those with shallow anterior chambers.

It is obvious from the numerous studies that a large effort is taking place in order to use CFD in order to understand the glaucoma pathophysiology[23] as well as to evaluate the therapeutic options for the disease.[24]

Vitreous

Anatomy

Figure 7: Vitreous humor

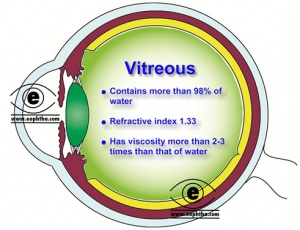
The vitreous is the translucent, colorless, gelatinous mass that fills the space between the lens of the eye and the retina lining the back of the eye. It is produced by certain retinal cells. It is of quite comparable composition to the cornea, but contains very few cells (mostly phagocytes which remove unwanted cellular debris in the visual field, as well as the hyalocytes of Balazs of the surface of the vitreous, which reprocess the hyaluronic acid), no blood vessels, and 98-99% of its volume is water (as opposed to 75% in the cornea) with salts, sugars, vitrosin (a type of collagen), a network of collagen type II fibers with the glycosaminoglycan hyaluronic acid, and also a broad range of proteins. Astoundingly, with so slight solid matter, it firmly holds the eye. However, the vitreous has a viscosity two to four times that of water, giving it a gelatinous consistency. (Figure 7 & 8)

Figure 8: Comparing viscosity of different materials.

It has a refractive index of 1.336. Although the vitreous is in contact with the retina and helps to keep it in place by pressing it against the choroid, it does not adhere to the retina, except in three places: around the anterior border of the retina; in the macula, and at the optic nerve disc.

Unlike the fluid in the frontal parts of the eye (aqueous humor) which is endlessly replenished, the gel in the vitreous chamber is stagnant. Therefore, if blood, cells or other byproducts of inflammation get into the vitreous, they will stay there. If the vitreous pulls away from the retina, it is known as a vitreous detachment. As we age, the vitreous often liquefies and may collapse. This is more likely to happen in eyes that are nearsighted. It can also occur after injuries to the eye or uveitis.

Pharmacokinetics

The current trend regarding management of disorders as macular edema, age-related macular degeneration, and diabetic retinopathy is intravitreal injection of pharmaceutical agents.[25][26] Many studies have conducted relating to the kinetics of such preparations aiming to elucidate the exact behavior of the agents inside the posterior segment.

  • A recent study tried to simulate dissolution of intravitreal triamcinolone acetonide suspensions in an anatomically precise rabbit eye model. In particular, the purpose of the study was to examine the impact of particle size on dissolution rate and residence of intravitreal suspension depots of triamcinolone acetonide with the assistance of CFD. The outcome of the study showed that since the size-independent limit was several-fold greater than the particle size of commercially available pharmaceutical triamcinolone suspensions, differences in particle size amongst such products are predicted to be irrelevant to their duration or performance.[27]
  • One even more recent study attempted to simulate drug distribution in the posterior segment of the eye after intravitreal injection and ocular implantation.[28] The outcome was that in intravitreal injection the drug concentration profile and its maximum value depended on parameters as the injection time, needle gauge, and penetration angle of the needle. In cases of implants insertion, locating the implant in posterior or anterior regions had an important consequence on drug concentrations. In addition, the shape of implant affected the concentration profile inside the eye. Analogous models could attribute to optimization of therapeutic benefits and reduction of the possibility of tissue toxicity.

Crystalline lens

Crystalline lens and and ciliary body are also structures that can be investigated by CFD. The lens is a transparent, biconvex structure in the eye that, in conjunction with the cornea, helps to refract light to be focused on the retina. The lens changes the focal distance of the eye so that it can focus on objects at various distances (accommodation).[29] Is also known as the aquula (Latin, a little stream, dim. of aqua, water) or crystalline lens.

In humans, the refractive power of the lens in its natural environment is roughly 18 diopters, about one-third of the eye's total power. The ciliary body is the circumferential tissue inside the eye composed of the ciliary muscle and ciliary processes. It is triangular in horizontal section and is coated by a double layer, the ciliary epithelium. This epithelium generates the aqueous humor.

  • Researchers tried to apply CFD in order to simulate heat exposure and damage to the eye lens. Lens epithelial samples were taken for analysis of catalase activities. Control lenses maintained their optical quality throughout the 14 days of the culture. Exposure to heat caused optical damage to the cultured lenses. The damage appeared earlier in the 6 hours exposure group and progressed from the lens anterior suture to its center. Optical damage was recovered in lenses exposed 1 hour to 39.5 degrees C, but the damage remained in the lens epithelial cells. This research group showed that exposure to heat in bakeries can cause damage to the eye lens and that the damage is dependent on the duration of exposure.[30]

Accommodation-Presbyopia

Another field of CFD application in ophthalmology is the investigation of accommodation. Accommodation acts like a reflex, but can also be consciously controlled. Mammals, birds and reptiles vary the optical power by changing the form of the elastic lens using the ciliary body (in humans up to 15 diopters). (Video 1) Fish and amphibians vary the power by changing the distance between a rigid lens and the retina with muscles.[31] The young human eye can change focus from distance to 7 cm from the eye in 350 milliseconds.

This remarkable alteration in focal power of the eye of approximately 12 diopters occurs as a consequence of a reduction in zonular tension induced by ciliary muscle contraction. The amplitude of accommodation declines with age (presbyopia).

  • A remarkable effort included a computational evaluation of the role of accommodation in pigmentary glaucoma.[32] Using an appropriate model, researchers confirmed that accommodation produces bowing of the posterior iris and the magnitude of the bowing is a strong function of the amount of accommodation.

Cataract fluidics

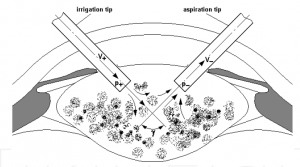
Figure 9. Flows during bimanual irrigation/aspiration.

Finally, a promising ground for CFD application could be the study of fluidics during phacoemulsification and in cataract surgery in general. (Figure 9)

  • After the development of a modified implantable collamer lens (ICL) with a central hole to improve aqueous humor circulation, researchers tried to investigate the fluid dynamic characteristics of aqueous humor in such a lens using CFD.[33]
  • Lately many researchers presented their work about application of CFD models during cataract operations depending on diverse parameters such as irrigation/aspiration, micro incisions, cataract grades or microcoaxial technique. [34][35][36]

Acknowledgements

Photo credits

  1. http://fenicsproject.org/featured/2011/pdesys.html
  2. http://www.chfc.ukw.de/forschung/forschungsprofessuren/cmi/forschung/computational-cardiology.html
  3. http://humorf1.info/aqueous-and-vitreous-humors/
  4. https://knowledge.autodesk.com/support/cfd/learn-explore/caas/CloudHelp/cloudhelp/2014/ENU/SimCFD/files/GUID-F6011744-B69B-47D0-BB2B-50E1BA8DC32D-htm.html
  5. http://www.glaucomacare.com.au/services/glaucoma/glaucoma-treatments
  6. http://www.eye7.in/glaucoma/laser-iridotomy/
  7. http://www.eophtha.com/eophtha/anatomy/anatomyofvitreous.html
  8. https://en.wikipedia.org/wiki/Viscosity
  9. http://cdn.intechopen.com/pdfs-wm/42693.pdf (significant work in open access article: Hydrodynamic Analysis and Irrigation Device Design for the Coaxial and Bimanual Phacoemulsification Techniques in Cataract Surgery by Yury Spirochkin

References

  1. ↑ Anderson, John D. Computational Fluid Dynamics: The Basics With Applications, Science/Engineering/Math, McGraw-Hill Science, 1995, ISBN 0070016852
  2. ↑ Hutmacher DW, Singh H. Computational fluid dynamics for improved bioreactor design and 3D culture. Trends Biotechnol 2008; 26: 166-72
  3. ↑ Wentzel JJ, Janssen E, Vos J, Schuurbiers JC, Krams R, et al. Extension of increased atherosclerotic wall thickness into high shear stress regions is associated with loss of compensatory remodeling. Circulation 2003; 108: 17-23.
  4. ↑ LaDisa JF Jr, Olson LE, Molthen RC, Hettrick DA, Pratt PF, et al. Alterations in wall shear stress predict sites of neointimal hyperplasia after stent implantation in rabbit iliac arteries. Am J Physiol Heart Circ Physiol 2005;288: H2465-75
  5. ↑ LaDisa JF Jr, Olson LE, Hettrick DA, Warltier DC, Kersten JR, et al. Axial stent strut angle influences wall shear stress after stent implantation: analysis using 3D computational fluid dynamics models of stent foreshortening. Biomed Eng Online 2005; 26; 4:59.
  6. ↑ Wrobel LC, Ginalski MK, Nowak AJ, Ingham DB, Fic AM. An overview of recent applications of computational modelling in neonatology. Philos Transact A Math Phys Eng Sci 2010;368:2817-34.
  7. ↑ Darquenne C, Harrington L, Prisk GK. Alveolar duct expansion greatly enhances aerosol deposition: a three-dimensional computational fluid dynamics study. Philos Transact A Math Phys Eng Sci 2009;367: 2333-46
  8. ↑ Farmakis TM, Soulis JV, Giannoglou GD, Zioupos GJ, Louridas GE. Wall shear stress gradient topography in the normal left coronary arterial tree: possible implications for atherogenesis. Curr Med Res Opin 2004;20:587-96.
  9. ↑ Winter FC. The lens and vitreous.Arch Ophthalmol 1967; 78: 229-55
  10. ↑ Holekamp NM. The vitreous gel: more than meets the eye. Am J Ophthalmol 2010;149: 32-6
  11. ↑ Sultan MB, Mansberger SL, Lee PP. Understanding the importance of IOP variables in glaucoma: a systematic review. Surv Ophthalmol 2009;54: 643-62.
  12. ↑ Kwon YH, Fingert JH, Kuehn MH, Alward WL. Primary open-angle glaucoma. N Engl J Med 2009; 360:1113-24.
  13. ↑ Alm A, Nilsson SF. Uveoscleral outflow-a review. Exp Eye Res 2009; 88:760-8.
  14. ↑ Tamm ER, Fuchshofer R. What increases outflow resistance in primary open-angle glaucoma? Surv Ophthalmol 2007; 52 Suppl 2:S101-4.
  15. ↑ Tan JC, Peters DM, Kaufman PL. Recent developments in understanding the pathophysiology of elevated intraocular pressure. Curr Opin Ophthalmol 2006;17: 168-74.
  16. ↑ Johnson DH. Trabecular meshwork and uveoscleral outflow models. J Glaucoma 2005; 14: 308-10.
  17. ↑ Fitt AD, Gonzalez G. Fluid Mechanics of the Human Eye: Aqueous Humour Flow in the Anterior Chamber Bulletin of Mathematical Biology 2006;68: 53–71
  18. ↑ Kumar S, Acharya S, Beuerman R, Palkama A. Numerical solution of ocular fluid dynamics in a rabbit eye: parametric effects. Ann Biomed Eng 2006; 34:530-44.
  19. ↑ Heys JJ, Barocas VH, Taravella MJ. Modeling passive mechanical interaction between aqueous humor and iris. J Biomech Eng 2001; 123:540-7.
  20. ↑ Ooi EH, Ng EY. Simulation of aqueous humor hydrodynamics in human eye heat transfer. Comput Biol Med 2008; 38: 252-62.
  21. ↑ Ethier CR, Read AT, Chan D. Biomechanics of Schlemm's canal endothelial cells: influence on F-actin architecture. Biophys J 2004;87: 2828-37.
  22. ↑ Kaji Y, Oshika T, Usui T, Sakakibara J. Effect of shear stress on attachment of corneal endothelial cells in association with corneal endothelial cell loss after laser iridotomy. Cornea 2005;24(8 Suppl):S55-S58.
  23. ↑ Kapnisis K, Doormaal MV, Ross Ethier C. Modeling aqueous humor collection from the human eye. J Biomech 2009; 42: 2454-7.
  24. ↑ Kotliar KE, Kozlova TV, Lanzl IM. Postoperative aqueous outflow in the human eye after glaucoma filtration surgery: biofluidmechanical considerations. Biomed Tech (Berl) 2009;54:14-22.
  25. ↑ Chiang A, Haller JA. Vitreoretinal disease in the coming decade. Curr Opin Ophthalmol 2010; 21:197-202.
  26. ↑ Ozkiris A. Anti-VEGF agents for age-related macular degeneration. Expert Opin Ther Pat 2010;20:103-18.
  27. ↑ Missel PJ, Horner M, Muralikrishnan R . Simulating dissolution of intravitreal triamcinolone acetonide suspensions in an anatomically accurate rabbit eye model. Pharm Res 2010; 27:1530-46.
  28. ↑ Jooybar E, Abdekhodaie MJ, Farhadi F, Cheng YL. Computational modeling of drug distribution in the posterior segment of the eye: effects of device variables and positions. Math Biosci. 2014 Sep;255:11-20.
  29. ↑ John Forrester, Andrew Dick, Paul McMenamin, William Lee. The Eye: Basic Sciences in Practice. 1996 London: W.B. Saunders Company Ltd. p. 28
  30. ↑ Sharon N, Bar-Yoseph PZ, Bormusov E, Dovrat A. Simulation of heat exposure and damage to the eye lens in a neighborhood bakery. Exp Eye Res2008; 87:49-55.
  31. ↑ Schachar RA. The mechanism of accommodation and presbyopia. International Ophthalmology Clinics 2006; 46: 39-61
  32. ↑ Heys JJ, Barocas VH. Computational evaluation of the role of accommodation in pigmentary glaucoma. Invest Ophthalmol Vis Sci 2003; 43:700-8.
  33. ↑ Kawamorita T, Uozato H, Shimizu K. Fluid dynamics simulation of aqueous humour in a posterior-chamber phakic intraocular lens with a central perforation. Graefes Arch Clin Exp Ophthalmol. 2012;250(6):935-9.
  34. ↑ Abouali O, Bayatpour D, Ghaffariyeh A, Ahmadi G. Simulation of flow field during irrigation/aspiration in phacoemulsification using computational fluid dynamics. J Cataract Refract Surg. 2011;37(8):1530-8.
  35. ↑ Narayanan A, Srinivasan U, Sankara Adhi P, Subbaiah S. An analysis to optimize the various aspects of micro incision phacoemulsification surgery on different grades of cataracts and the micro incision IOL implantation. Poster presented in XXXI annual congress of ESCRS, Amsterdam, October 2013
  36. ↑ Subbiah S, Narayanan A. Computational Fluid Dynamics Simulation of Human Eye Anterior Chamber Fluidics During Regular and Microcoaxial Phacoemulsification. Poster presented in ASCRS congress, April 2014.
Retrieved from "https://eyewiki.org/w/index.php?title=Computational_Fluid_Dynamics_(CFD)_in_Ophthalmology&oldid=113500"
Powered by MediaWiki
Powered by Canasta
Powered by Semantic MediaWiki